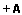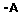Resumo: Os conceitos de recursividade e dispersividade para sistemas dinâmicos em espaços métricos estão relacionados com estabilidade de Poisson, pontos não-dispersivos, instabilidade de Poisson e pontos-dispersivos. No presente trabalho será exposto uma extensão destes conceitos para ações de semigrupos em espaços admissíveis. Apresentaremos os conceitos de prolongamento, conjuntos limites prolongacionais, estabilidade de Poisson, pontos não-dispersivos, instabilidade de Poisson e pontos-dispersivos para ações de semigrupos. Provaremos a principal propriedade de um ponto não-dispersivo, ou seja, o ponto é não-dispersivo se, e somente se, este ponto pertence ao seu conjunto limite prolongacional e mostraremos quais condições para que a ação é dispersiva se, e somente se, para todo ponto do espaço topológico, o prolongamento deste ponto é igual a sua órbita e não existem pontos quase periódicos. Em seguida, apresentaremos algumas aplicações para sistemas de controle e fibrados
Abstract: The recursive and dispersive concepts for dynamical systems in metric spaces are related to Poisson stability, non-wandering points, Poisson instability and dispersive points. The present thesis extends these concepts to semigroup actions on admissible spaces. We present the concepts of prolongation, prolongational limit sets, Poisson stability, non-wandering points, Poisson instability and dispersive points for semigroups actions. Prove the main property of a non-dispersive point, i.e., the point is non-dispersive if, and only if, this point belongs to the whole prolongational limit and show conditions for which the action is dispersive if, and only if, for every point of the topological space, the prolongation of this point is equal to its orbit and there are not almost periodic points. We also present some applications to control systems and fiber bundles |