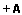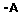Resumo: Neste trabalho, abordaremos o problema de encontrar a cardinalidade mínima de um código de cobertura no espaço finito de Hamming. Esta cardinalidade mínima será dada pela função Kq(n, R), e apresentaremos valores exatos e aproximações para algumas classes desta função através da teoria dos códigos de cobertura. Estas construções podem ser feitas através de argumentos combinatórios e em algumas delas são usadas ferramentas algébricas, propriedades de corpos finitos, a teoria aditiva dos números, construções matriciais. Tais construções serão úteis na obtenção de limites superiores. Por outro lado, métodos utilizando s-sobrejetividade e partição de matrizes serão de grande utilidade na obtenção de alguns limites inferiores para algumas classes da função Kq(n, R)
Abstract: In this work, we will approach the issue to find the minimum cardinality of a covering code in the Hamming finite space. This minimum cardinality will be given by the function Kq(n, R), and we will introduce exact values and approximations to some classes of these functions through the covering codes theory. These constructions can be done through combinatory arguments and in some of them we will use algebraic tools using finite fields properties, the numbers addictive theory, constructions using matrixes. These constructions will be very useful to get upper bounds. on the other hand methods using the s-surjective and matrixes partition will be very useful to get some lower bounds to some classes of the function Kq(n, R) |