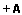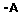Resumo: Neste trabalho, a partir das formas da condição inicial e da função de Green, identificamos o comportamento assintótico temporal de soluções das equações de difusão e de Schrödinger usuais e fracionárias. As condições iniciais empregadas variam desde as situações localizadas (cauda curta) até aquelas pouco localizadas (cauda longa). Em contraste com o caso de cauda curta, a presença de cauda longa para a condição inicial atrapalha o processo difusivo, no sentido de tornar o alargamento do pacote inicial mais lento. Esses fatos são conseqüência de funções de Green que sofrem um alargamento progressivo com o tempo. Isso, para tempos longos, faz com que a condição inicial fique bastante concentrada em relação a função de Green, e a partir daí fizemos nossas aproximações. Essas estimativas, independentemente da forma concreta da função de Green, podem ser expressas, essencialmente, em termos dos momentos da condição inicial e de derivadas de mesma ordem da função de Green.
Abstract: In this work, from the initial conditions and the Green functions, we identified the time asymptotic behavior of solutions of the usual diffusion and Schrödinger equations, as well as of the spatial fractional ones. The initial conditions employed here vary from localized (short tail) ones; to not much localized (long tail) ones. In contrast with the short tail case, the presence of the long tail for the initial condition disturbs the diffusive process, in a such way the spreading of the initial packet becomes slower. These facts are a consequence of the Green functions that progressively spreads with time. For long times, this behavior leads to a very wide Green function in comparison with the initial condition. From this we performed our approximations. These approximations, independently of the specific form of the Green function, can essentially be written in terms of the moments of the initial condition and the derivatives of the Green function. |