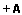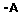Resumo: Analisamos a resposta dinâmica da influência dos íons presentes em um cristal líquido submetido a uma voltagem externa do tipo degrau. A evolução temporal do sistema é investigada resolvendo a equação da continuidade, para as densidades superficiais e as volumétricas, levando em conta a corrente de "drift" e de difusão. Também foi investigada a influência da adsorção nas interfaces onde o fenômeno transitório pode ocorrer. No limite de pequenas amplitudes do potencial aplicado, para o qual a equação do problema pode ser linearizada, obtemos uma solução analítica para as densidades superficiais e volumétricas de íons. Obtemos também, neste limite, o tempo de relaxação para o fenômeno transitório. Além disso, analisamos também se o conceito de impedância elétrica pode ser útil para analisar uma célula eletrolítica submetida a uma diferença de potencial. Para este último, usamos uma função simples dependente do tempo. Em todas as situações, resolvemos numericamente a equação diferencial que governa o fenômeno de re-distribuição de íons e comparamos com os resultados obtidos analiticamente.
Abstract: We analyze the dynamical response of the influence of the ions present in a liquid crystal submitted to a steplike external voltage. The time evolution of the system is investigated by solving the continuity equation, for the bulk and surface densities, taking into account the current of drift and diffusion. As well as, the influence of the adsorption at the interfaces on the transient phenomena occurring is investigated. In the limit of small amplitude of the applied potential, where the equation of problem can be linearized, we obtain an analytical solution for the bulk and surface densities of the ions. We also obtain, in this limit, the relaxation time for the transient phenomena. In addition, we analyze too if the concept of electrical impedance can be useful for to analyze a electrolytic cell submitted to a external voltage. For this last, we assume a simple harmonic function of the time. In all situations, the analysis is formed by solving numerically the differential equations governing the phenomenon of the redistribution of the ions and comparing the results with the ones obtained analytically. |